Lesson 3: Adding and Subtracting Fractions
/en/fractions/comparing-and-reducing-fractions/content/
Adding and subtracting fractions
In the previous lessons, you learned that a fraction is part of a whole. Fractions show how much you accept of something, similar 1/ii of a tank of gas or 1/three of a loving cup of water.
In real life, you might demand to add together or subtract fractions. For example, take yous ever walked 1/2 of a mile to work and so walked another 1/ii mile back? Or tuckered ane/four of a quart of gas from a gas tank that had 3/4 of a quart in it? You probably didn't recollect nearly it at the time, only these are examples of adding and subtracting fractions.
Click through the slideshow to larn how to set up add-on and subtraction problems with fractions.
-

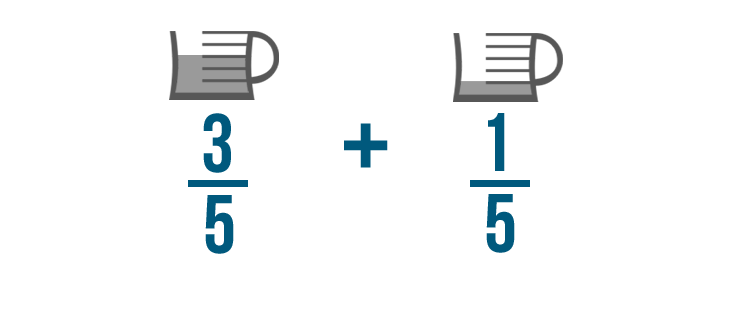
Let'due south imagine that a cake recipe tells you to add 3/5 of a loving cup of oil to the batter.
-
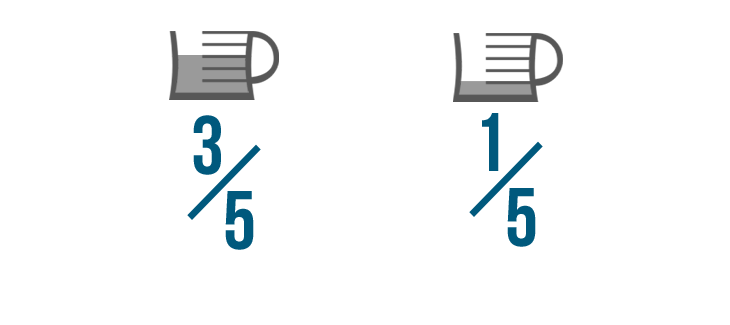
Yous also demand 1/5 of a cup of oil to grease the pan. To see how much oil you'll demand total, y'all can add these fractions together.
-

When you add fractions, you lot just add the height numbers, or numerators .
-
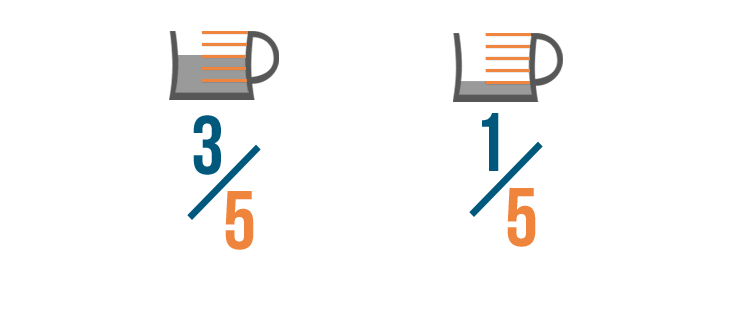
That's because the bottom numbers, or denominators , prove how many parts would make a whole.
-

We don't desire to change how many parts make a whole cup (v). We just want to detect out how many parts nosotros need total.
-

So nosotros just need to add the numerators of our fractions.
-
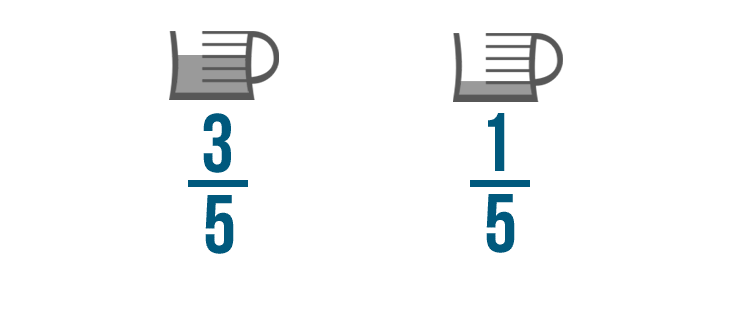
Nosotros can stack the fractions so the numerators are lined upwardly. This volition make information technology easier to add together them.
-
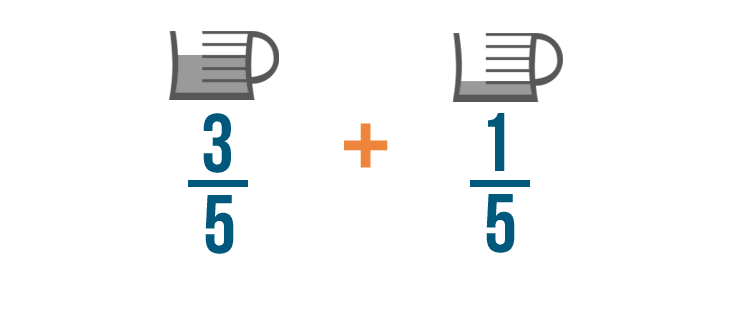
And that's all we take to practice to set an add-on example with fractions. Our fractions are now ready to exist added.
-

We'll do the same affair to set up a subtraction example. Permit'southward say you had iii/4 of a tank of gas when yous got to work.
-

If you lot apply 1/4 of a tank to drive home, how much will yous have left? We can subtract these fractions to find out.
-
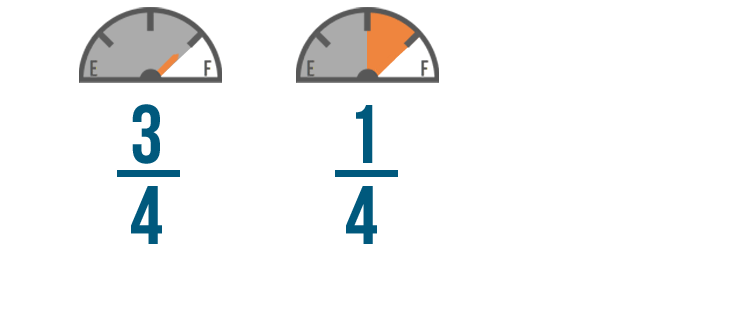
Just like when we added, we'll stack our fractions to keep the numerators lined upwardly.
-

This is considering we desire to subtract 1 function from three parts.
-
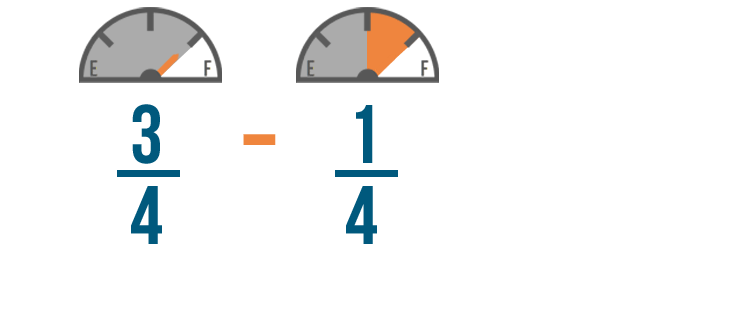
Now that our example is gear up, we're gear up to subtract!
-

Try This!
Endeavour setting up these add-on and subtraction issues with fractions. Don't try solving them nevertheless!
Y'all run 4/10 of a mile in the morning time. Afterward, you run for 3/10 of a mile.
Y'all had 7/8 of a stick of butter and used 2/viii of the stick while cooking dinner.
Your gas tank is ii/5 full, and you put in some other 2/5 of a tank.
Solving add-on issues with fractions
Now that we know how to write improver issues with fractions, let's practice solving a few. If you can add whole numbers, you're ready to add together fractions.
Click through the slideshow to learn how to add fractions.
-
Permit'southward continue with our previous example and add these fractions: three/5 of cup of oil and 1/5 of a cup of oil.
-
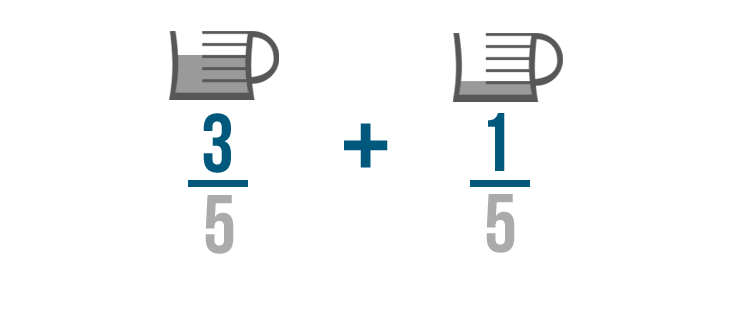
Remember, when we add together fractions, we don't add together the denominators.
-
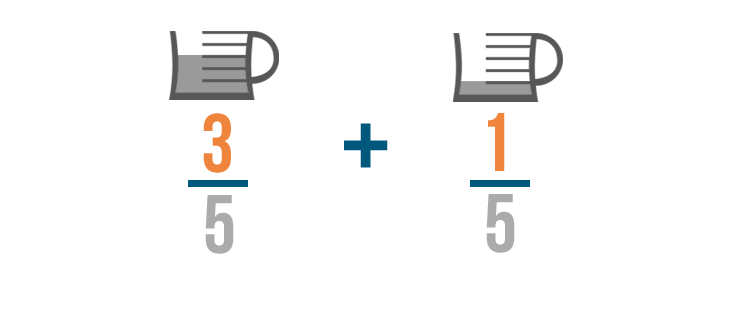
This is because we're finding how many parts we need full. The numerators show the parts we need, then we'll add together three and 1.
-
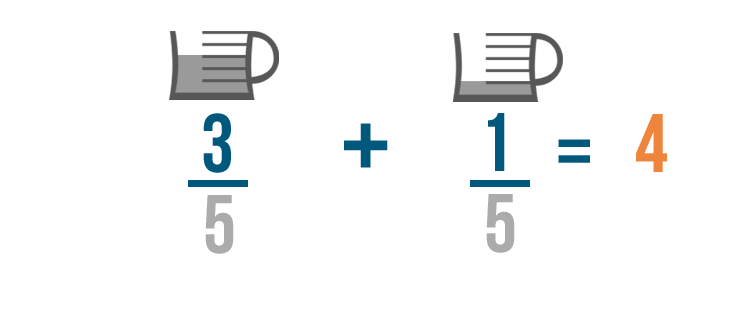
iii plus 1 equals 4. Brand certain to line up the iv with the numbers you just added.
-
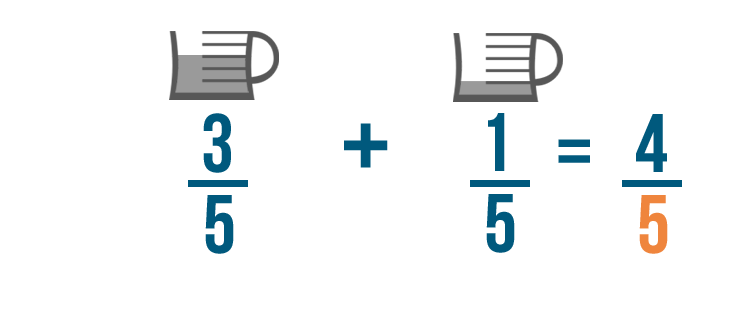
The denominators will stay the same, and then nosotros'll write 5 on the lesser of our new fraction.
-
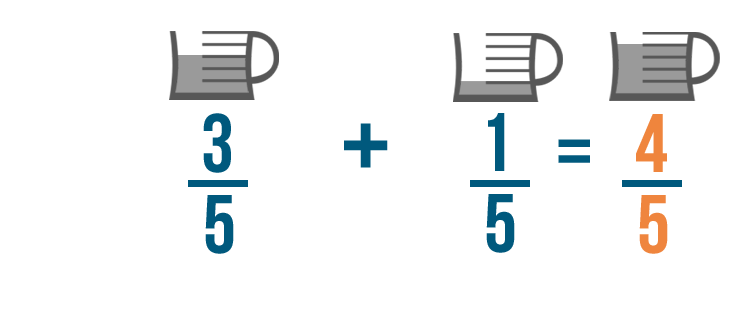
3/5 plus one/5 equals 4/5. And then you'll demand four/v of a cup of oil total to brand your cake.
-
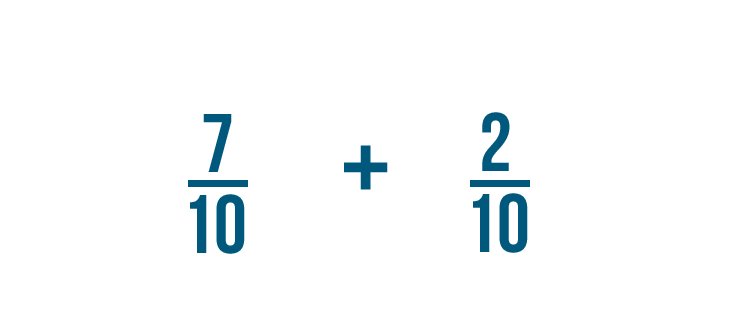
Let'southward endeavor some other example: seven/x plus two/x.
-

Just similar before, we're only going to add together the numerators. In this example, the numerators are 7 and 2.
-
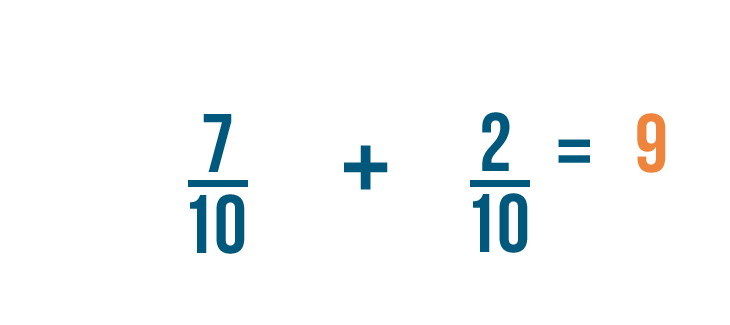
7 plus two equals 9, and then we'll write that to the right of the numerators.
-
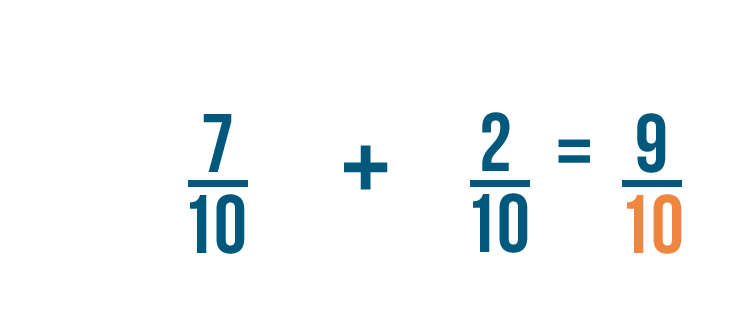
Just like in our earlier example, the denominator stays the same.
-
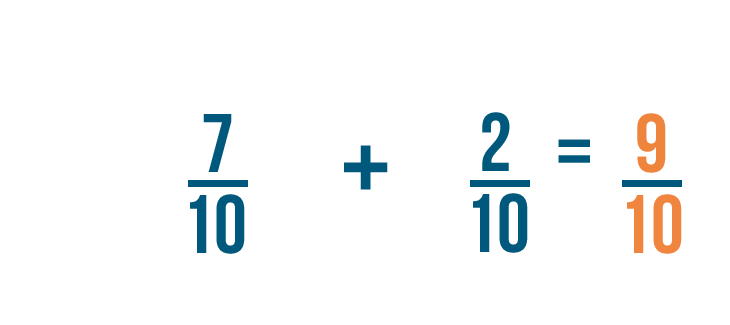
So 7/ten plus two/10 equals nine/10.
-

Try This!
Effort solving some of the addition issues beneath.
Solving subtraction bug with fractions
Subtracting fractions is a lot similar regular subtraction. If you tin can subtract whole numbers, yous can subtract fractions also!
Click through the slideshow to larn how to subtract fractions.
-

Let'southward use our before example and subtract 1/4 of a tank of gas from iii/4 of a tank.
-
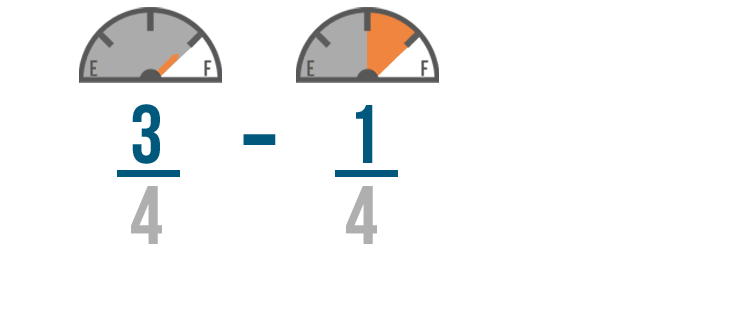
Just similar in improver, nosotros're not going to change the denominators.
-
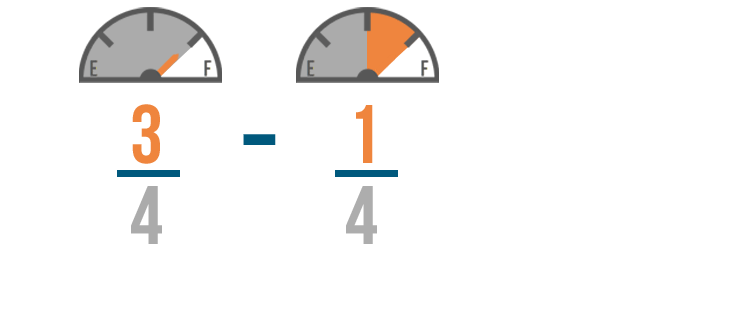
We don't want to change how many parts make a whole tank of gas. We just want to know how many parts we'll have left.
-

We'll offset by subtracting the numerators. 3 minus 1 equals ii, so we'll write 2 to the right of the numerators.
-
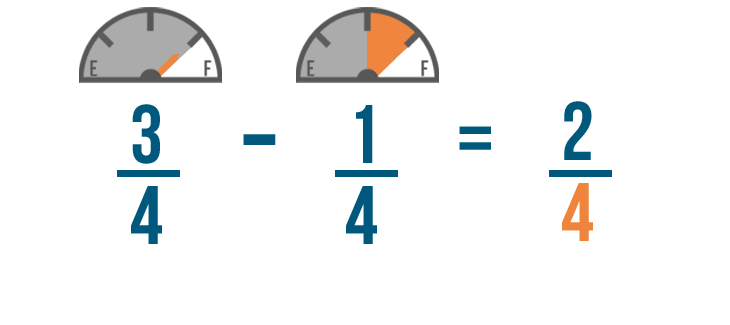
Just like when we added, the denominator of our answer will be the aforementioned every bit the other denominators.
-
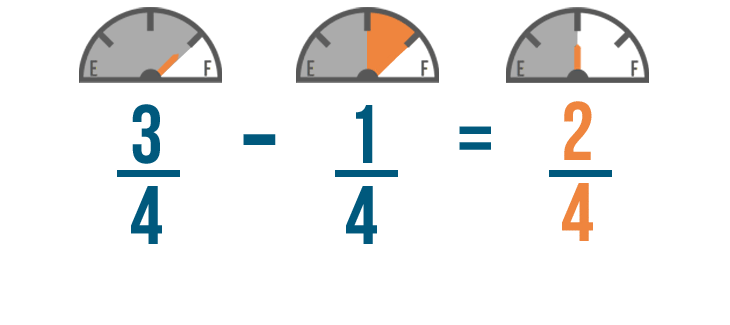
And so 3/4 minus ane/4 equals 2/4. You lot'll have ii/4 of a tank of gas left when y'all go home.
-
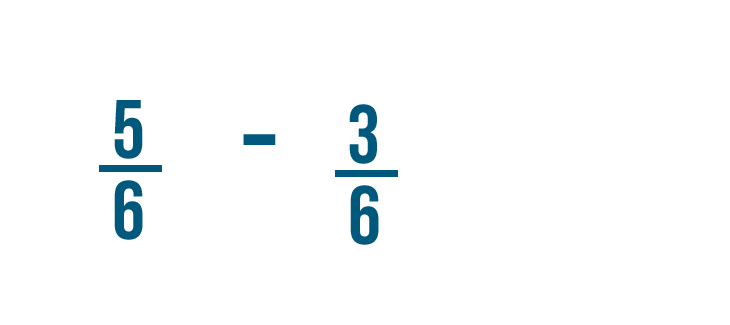
Let's effort solving some other problem: 5/6 minus iii/half-dozen.
-
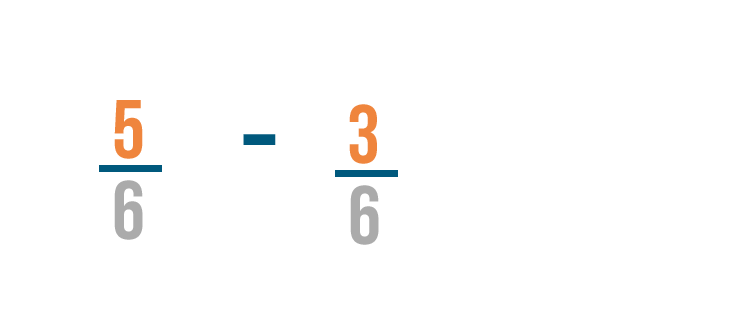
We'll start by subtracting the numerators.
-
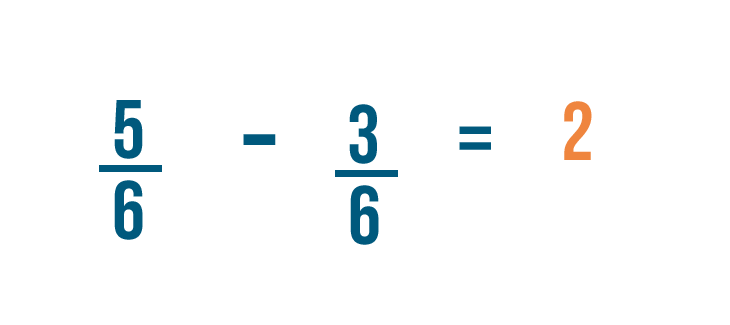
five minus three equals 2. So we'll put a 2 to the right of the numerators.
-

As usual, the denominator stays the aforementioned.
-
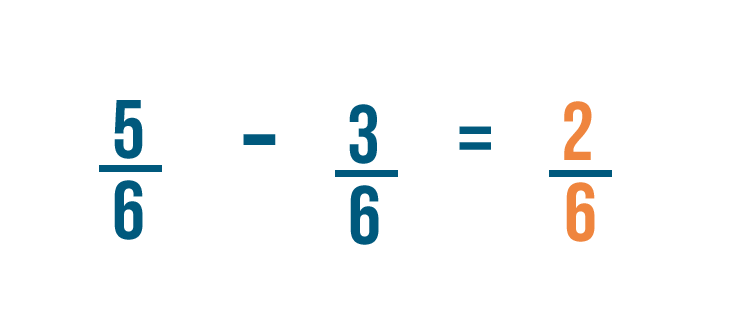
So v/half-dozen minus 3/6 equals two/vi.
-

Endeavor This!
Try solving some of the subtraction issues below.
After you lot add together or subtract fractions, you may sometimes have a fraction that can exist reduced to a simpler fraction. As you learned in Comparison and Reducing Fractions, it'south always all-time to reduce a fraction to its simplest grade when you can. For example, 1/four plus i/4 equals 2/iv. Because 2 and 4 tin both be divided two, we can reduce 2/4 to one/2.
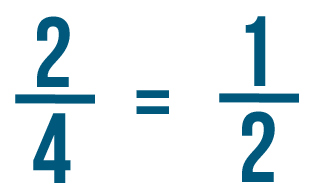
Adding fractions with different denominators
On the terminal page, we learned how to add fractions that take the same denominator, like ane/4 and three/iv. Only what if you needed to add fractions with different denominators? For example, our cake recipe might say to blend one/4 loving cup of milk in slowly and then dump in another 1/3 of a cup.
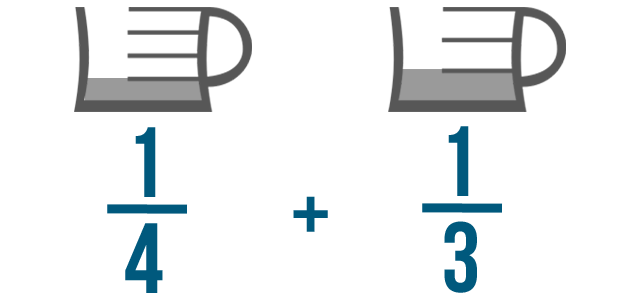
In Comparing and Reducing Fractions, we compared fractions with a different bottom number, or denominator. We had to change the fractions and then their denominators were the aforementioned. To practise that, we institute the lowest common denominator, or LCD.
Nosotros can only add or decrease fractions if they have the same denominators. So we'll demand to detect the lowest common denominator before nosotros add or subtract these fractions. One time the fractions take the same denominator, we can add together or subtract as usual.
Click through the slideshow to larn how to add fractions with different denominators.
-

Allow's add 1/4 and one/3.
-
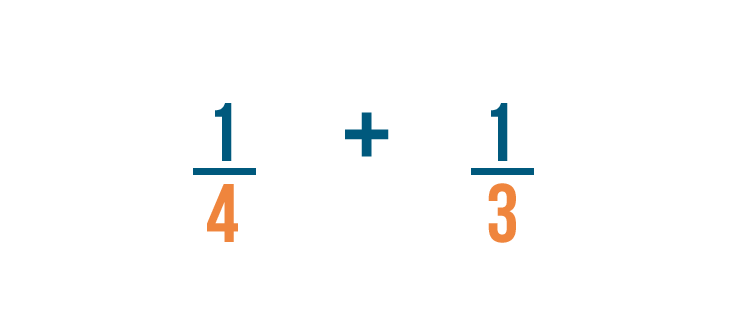
Before we tin add these fractions, we'll need to change them so they have the same denominator.
-
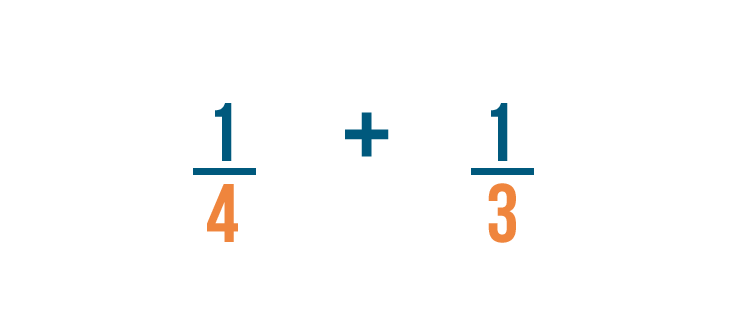
To practise that, we'll have to find the LCD, or lowest common denominator, of four and 3.
-
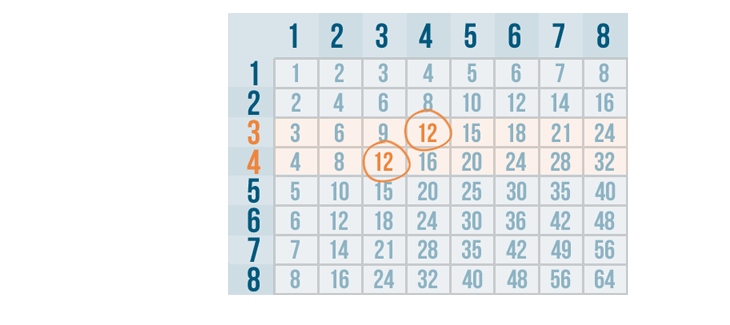
It looks like 12 is the smallest number that tin be divided by both three and four, and so 12 is our LCD.
-
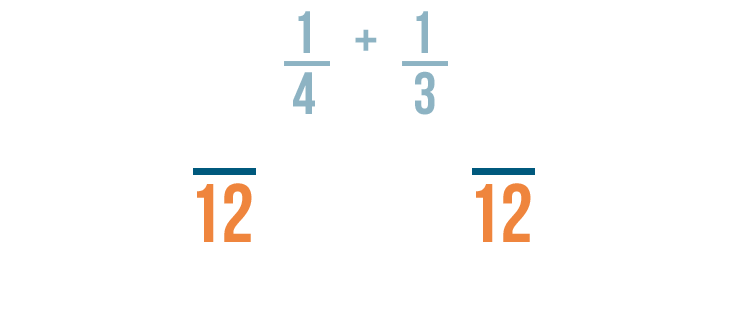
Since 12 is the LCD, it will be the new denominator for our fractions.
-
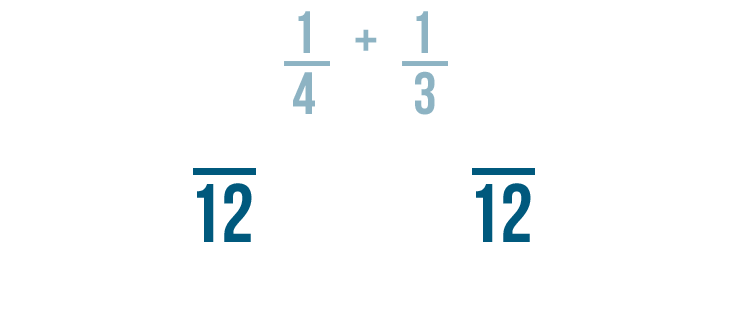
Now we'll change the numerators of the fractions, just similar we changed the denominators.
-
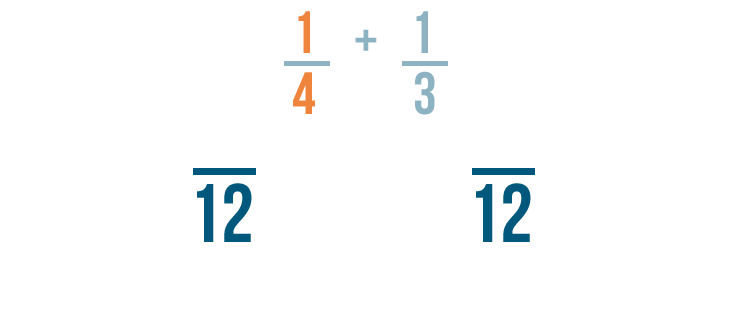
Get-go, allow'due south look at the fraction on the left: one/four.
-
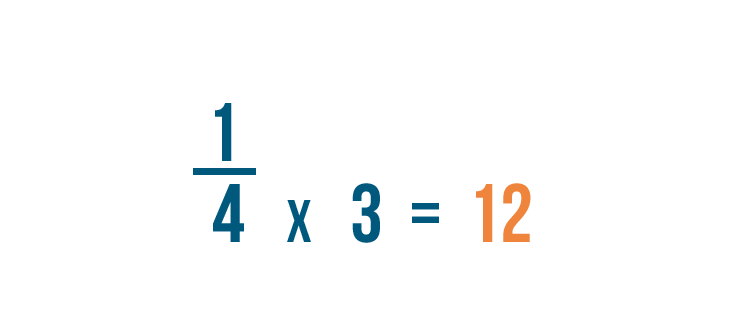
To alter 4 into 12, nosotros multiplied information technology past 3.
-
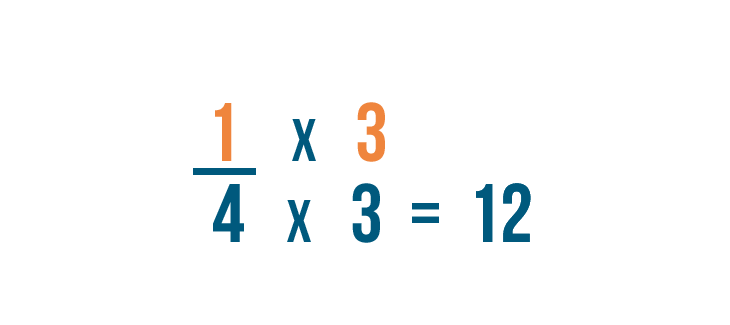
Since the denominator was multiplied by 3, we'll as well multiply the numerator by 3.
-
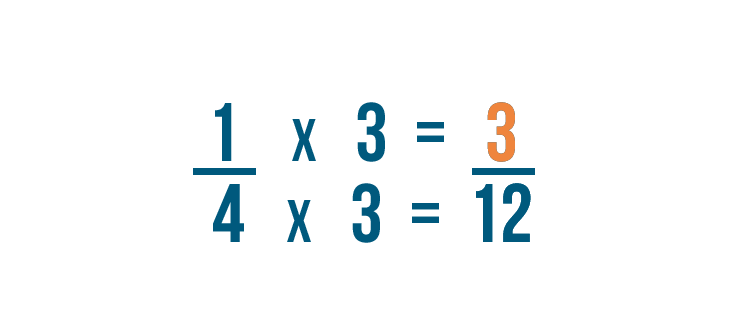
one times iii equals 3.
-
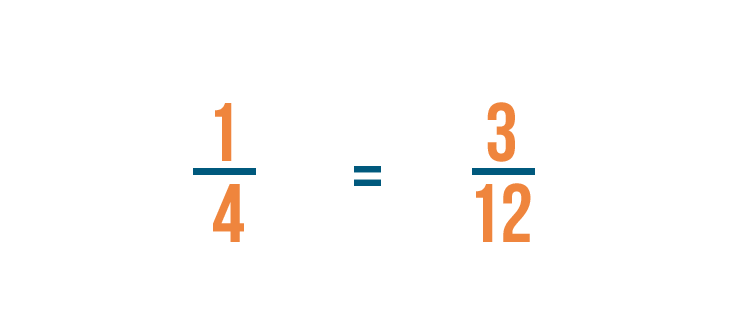
one/iv is equal to 3/12.
-
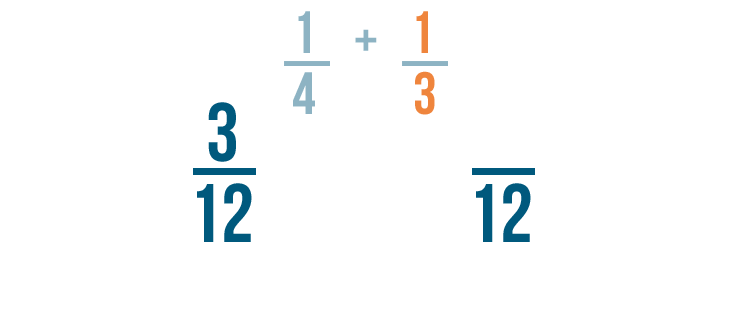
Now let'south await at the fraction on the right: 1/three. We changed its denominator to 12 as well.
-
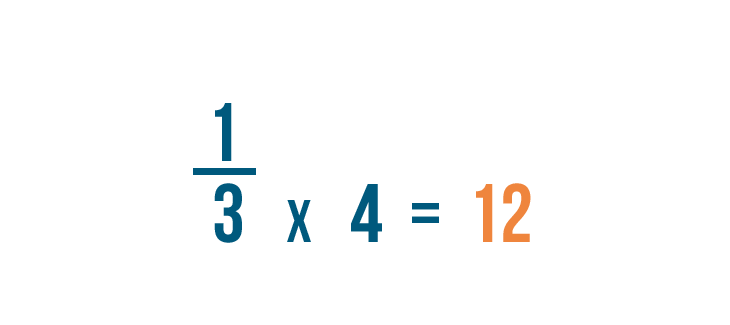
Our one-time denominator was 3. We multiplied information technology by four to get 12.
-

Nosotros'll besides multiply the numerator past 4. i times iv equals iv.
-

Then 1/iii is equal to 4/12.
-
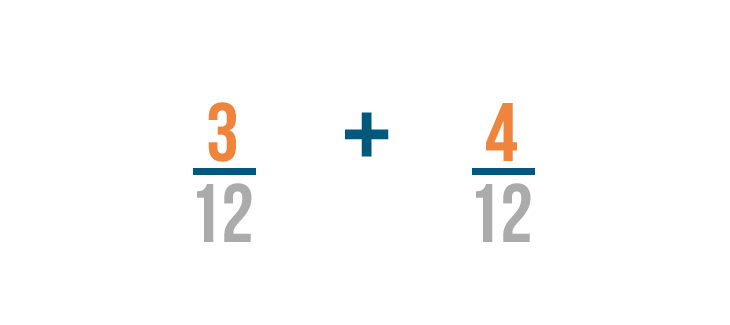
Now that our fractions have the same denominator, we can add them like we unremarkably do.
-
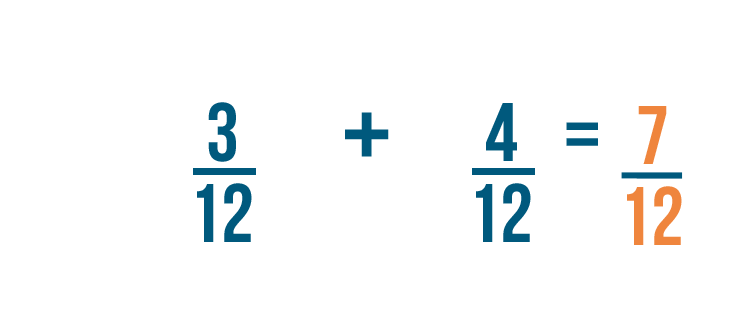
3 plus 4 equals 7. Equally usual, the denominator stays the same. So 3/12 plus 4/12 equals 7/12.
-

Endeavour This!
Try solving the addition problems below.
Subtracting fractions with different denominators
Nosotros but saw that fractions can merely exist added when they take the aforementioned denominator. The same thing is true when nosotros're subtracting fractions. Before nosotros tin can subtract, we'll accept to modify our fractions so they have the same denominator.
Click through the slideshow to learn how to subtract fractions with dissimilar denominators.
-
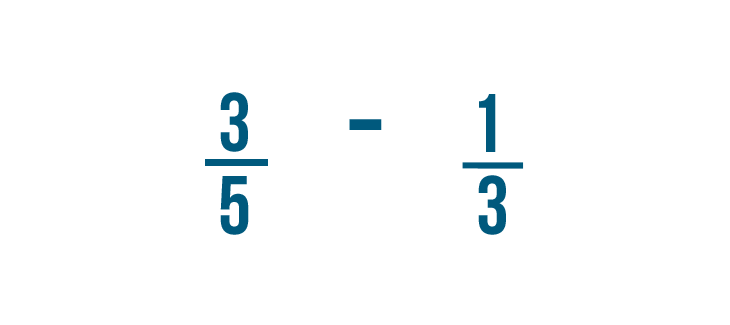
Permit'southward try subtracting one/3 from 3/5.
-
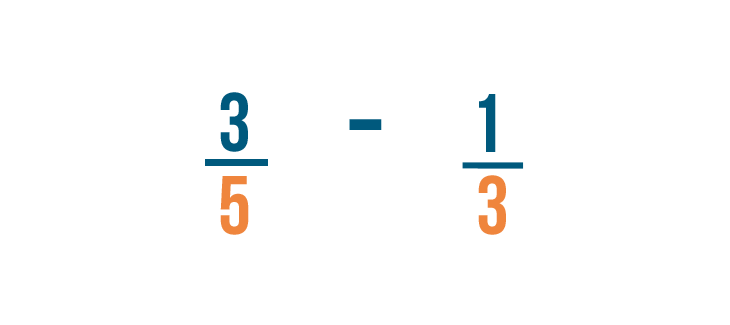
Start, nosotros'll modify the denominators of both fractions to be the same by finding the lowest common denominator.
-
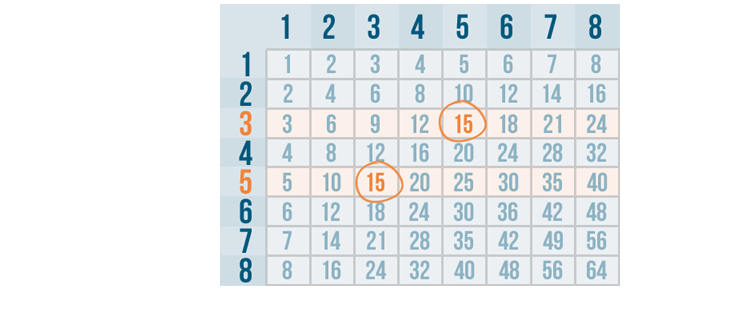
Information technology looks like xv is the smallest number that can exist divided evenly by three and 5, then 15 is our LCD.
-

Now we'll change our commencement fraction. To change the denominator to xv, we'll multiply the denominator and the numerator by 3.
-
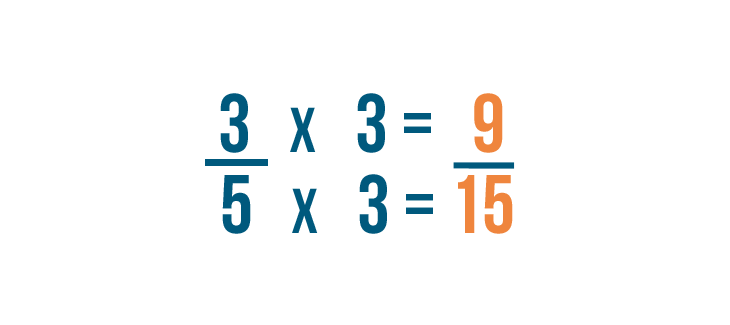
5 times 3 equals 15. And so our fraction is now nine/fifteen.
-

At present let's change the second fraction. To alter the denominator to fifteen, we'll multiply both numbers past 5 to get 5/15.
-
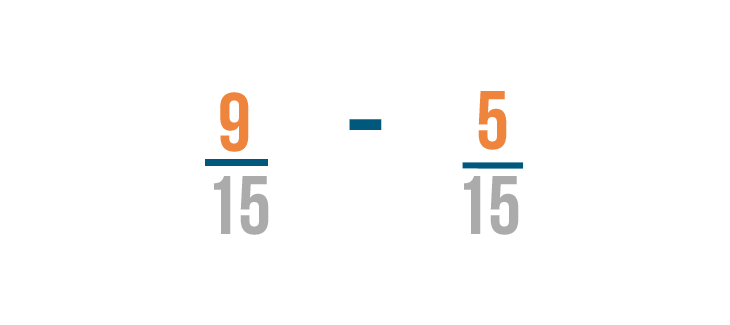
Now that our fractions have the aforementioned denominator, we tin can decrease like nosotros normally practise.
-

9 minus 5 equals 4. As always, the denominator stays the same. Then 9/15 minus five/15 equals 4/xv.
-

Try This!
Try solving the subtraction problems beneath.
Adding and subtracting mixed numbers
Over the last few pages, you lot've practiced adding and subtracting dissimilar kinds of fractions. But some problems will need one extra pace. For example, tin you add the fractions below?
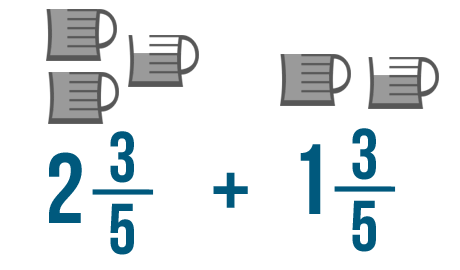
In Introduction to Fractions, you lot learned nearly mixed numbers. A mixed number has both a fraction and a whole number. An example is two 1/2, or two-and-a-one-half. Another way to write this would be v/2, or 5-halves. These 2 numbers look unlike, but they're actually the aforementioned.
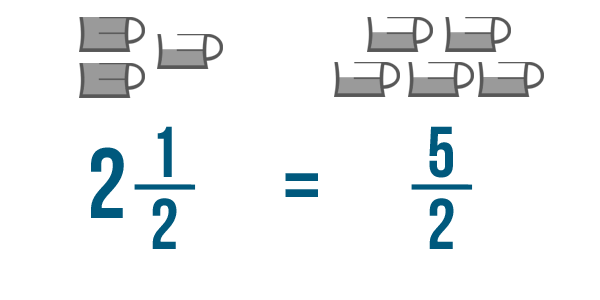
5/2 is an improper fraction. This just means the top number is larger than the bottom number. Even though improper fractions look foreign, y'all can add and subtract them just like normal fractions. Mixed numbers aren't like shooting fish in a barrel to add, then you'll have to convert them into improper fractions start.
-

Let'southward add these 2 mixed numbers: 2 three/5 and one 3/five.
-
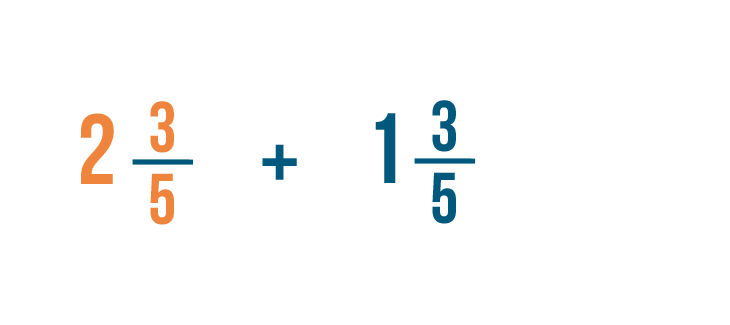
We'll need to catechumen these mixed numbers to improper fractions. Permit's first with 2 iii/5.
-
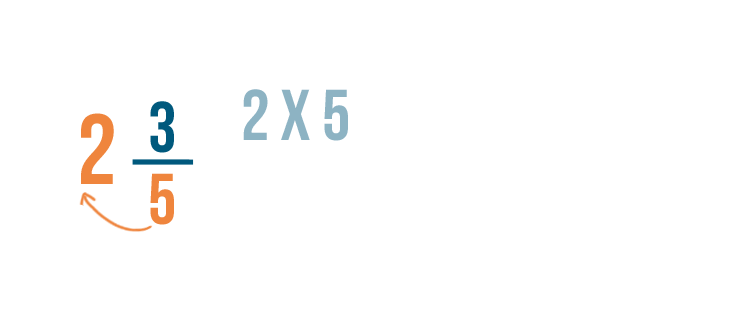
As you learned in Lesson 2, we'll multiply the whole number, ii, by the bottom number, 5.
-

ii times 5 equals ten.
-
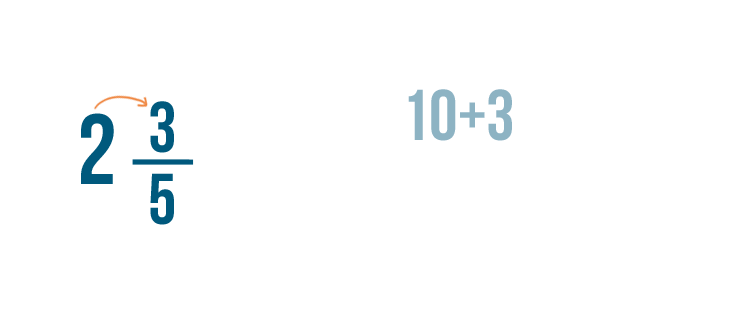
Now, let's add 10 to the numerator, 3.
-
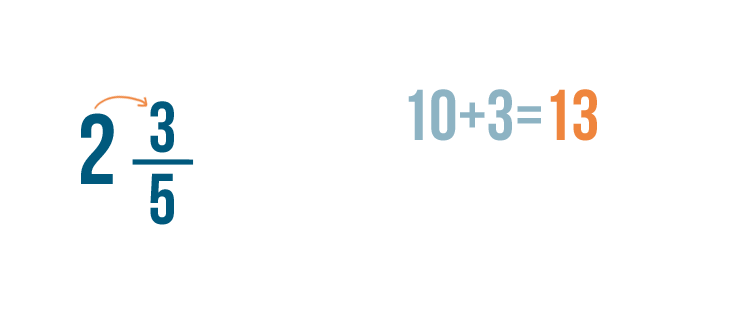
10 + 3 equals 13.
-
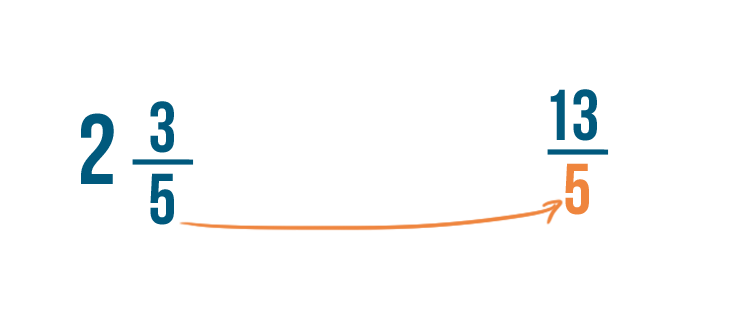
Simply like when you add fractions, the denominator stays the same. Our improper fraction is 13/5.
-
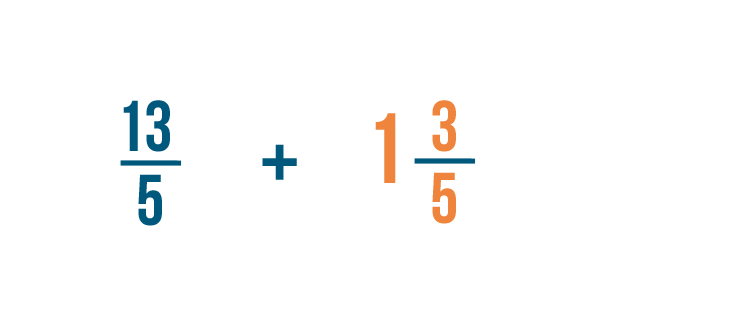
Now we'll need to convert our second mixed number: ane 3/5.
-

Outset, we'll multiply the whole number by the denominator. 1 10 v = v.
-
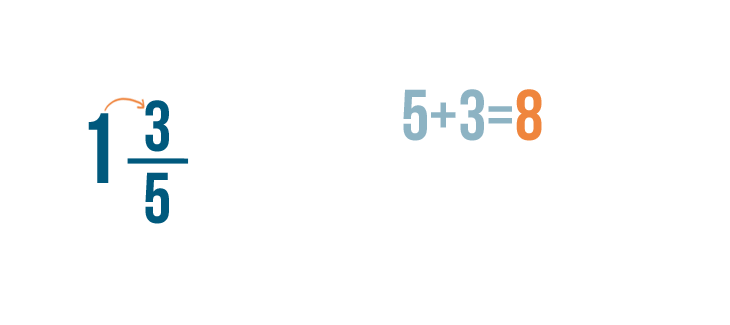
Adjacent, we'll add 5 to the numerators. 5 + 3 = 8.
-

Simply like concluding time, the denominator remains the same. So we've changed 1 3/v to viii/5.
-
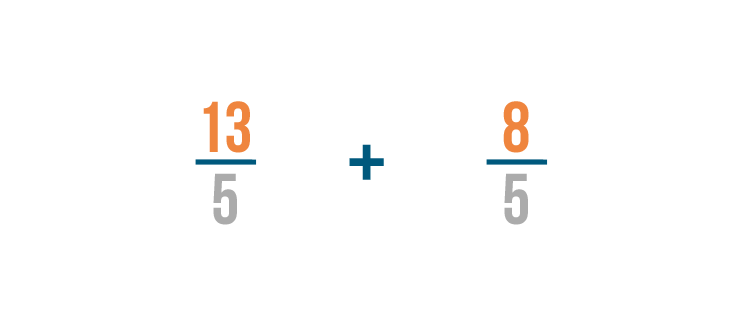
Now that we've inverse our mixed numbers to improper fractions, we tin can add like we commonly exercise.
-
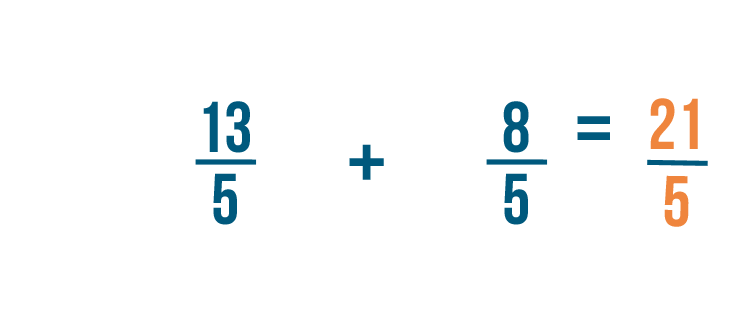
13 plus 8 equals 21. As usual, the denominator will stay the aforementioned. Then 13/5 + eight/5 = 21/5.
-
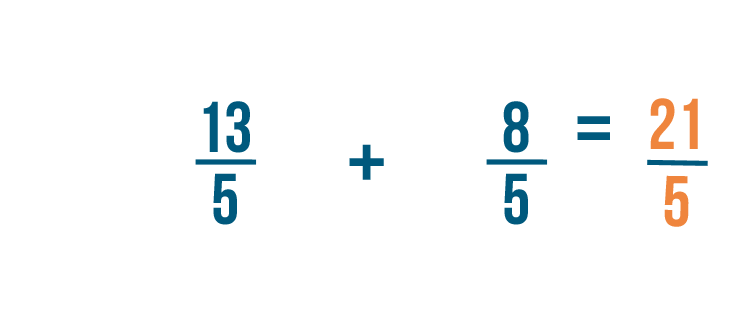
Because we started with a mixed number, let's convert this improper fraction back into a mixed number.
-
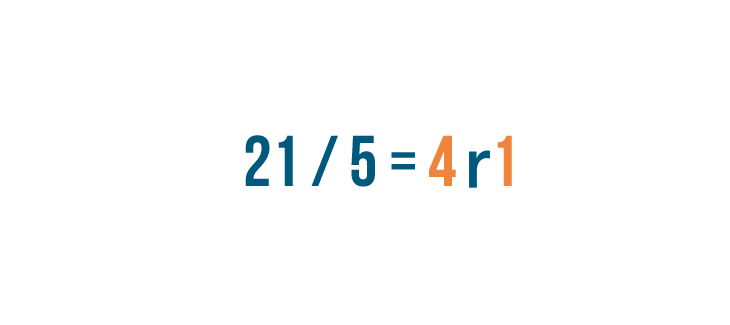
As you learned in the previous lesson, dissever the top number by the lesser number. 21 divided by five equals iv, with a remainder of ane.
-
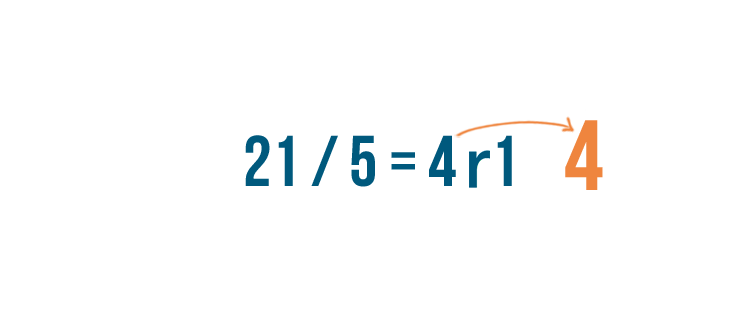
The answer, four, will become our whole number.
-
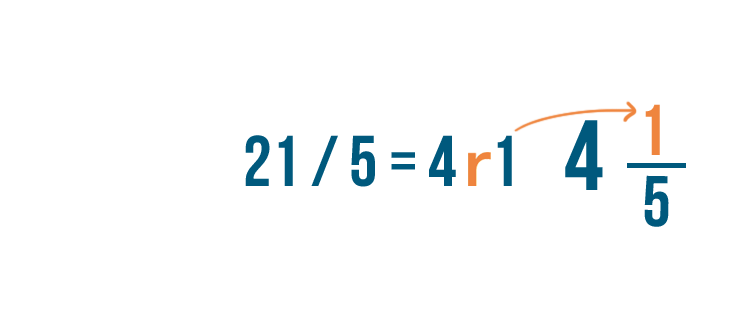
And the remainder, 1, volition go the numerator of the fraction.
-
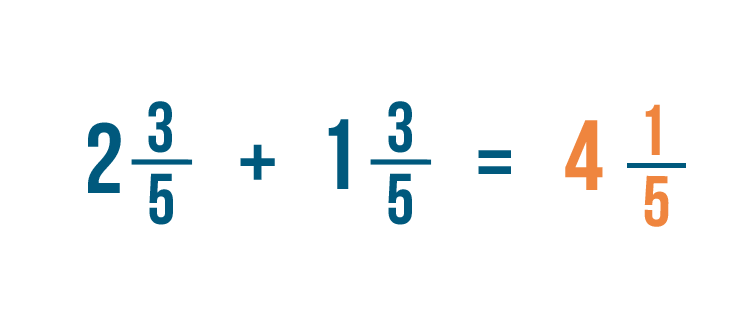
So 2 3/v + 1 3/five = 4 1/5.
-

/en/fractions/multiplying-and-dividing-fractions/content/





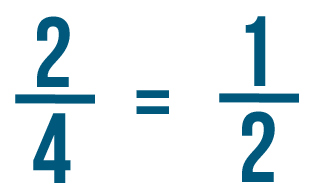
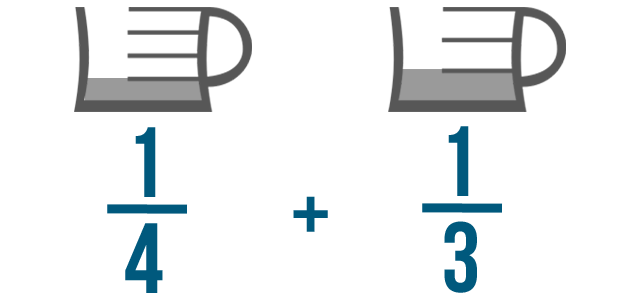
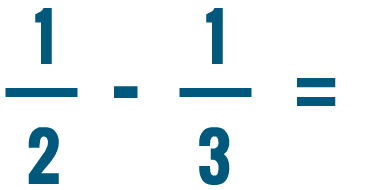
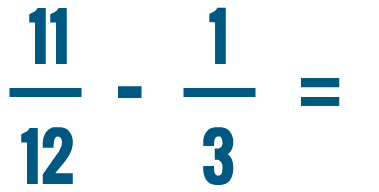

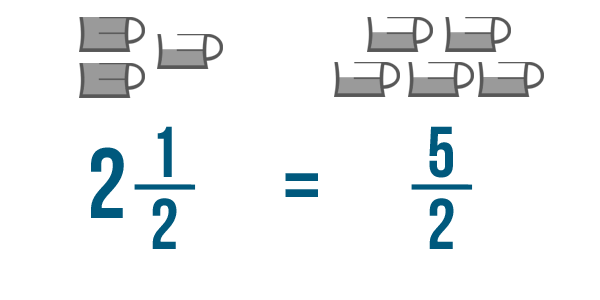





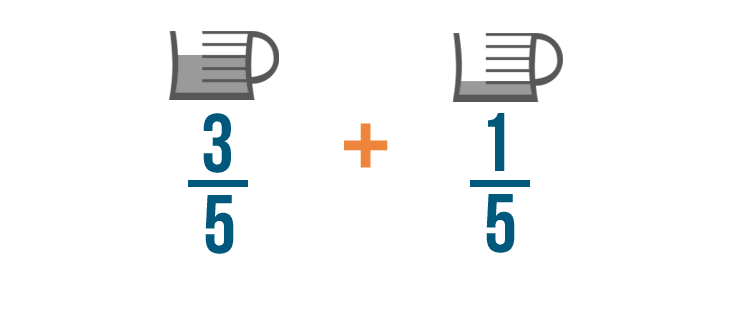


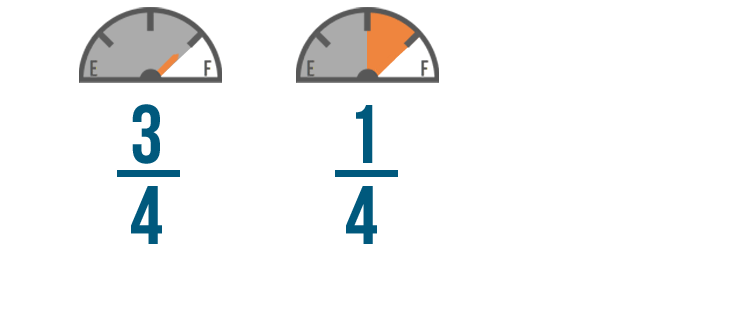

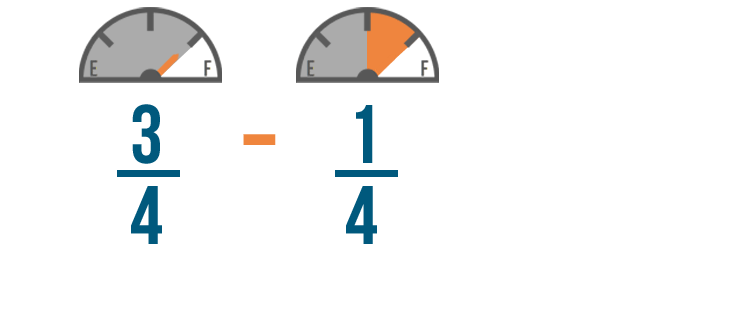







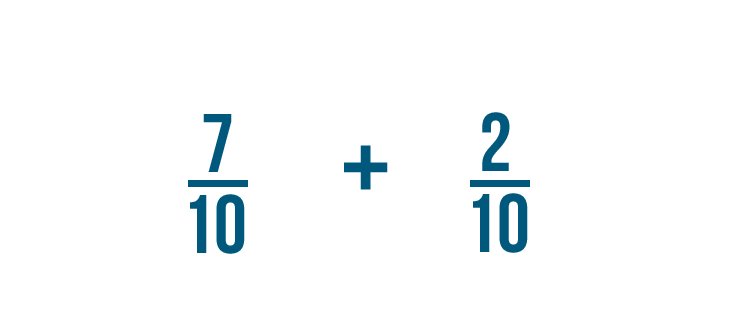

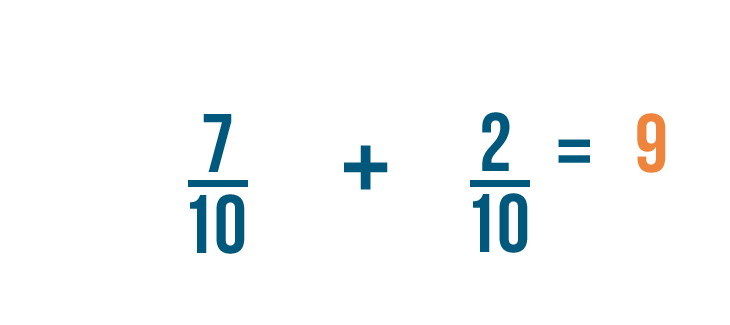
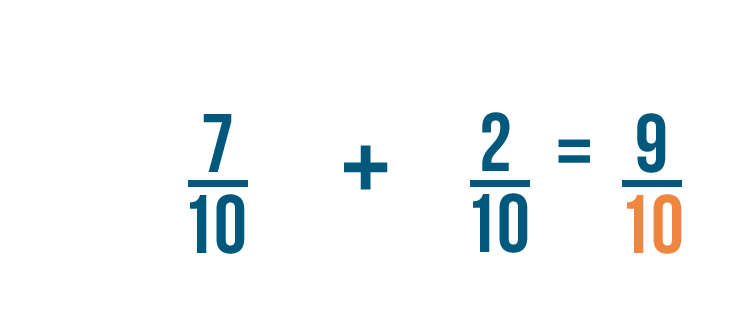
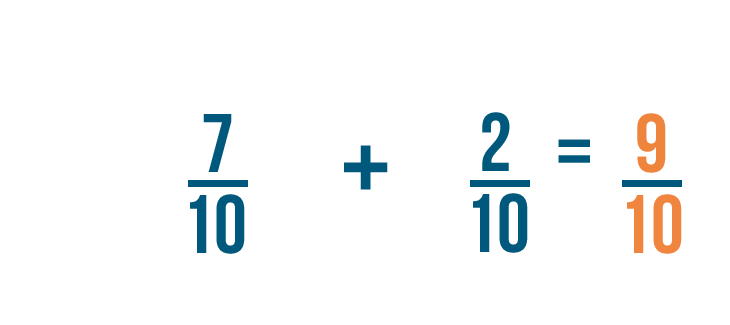

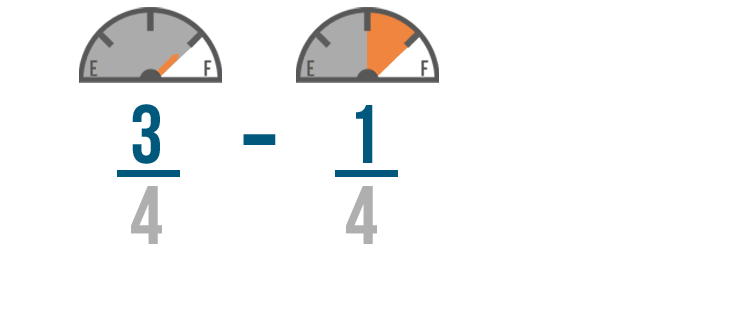
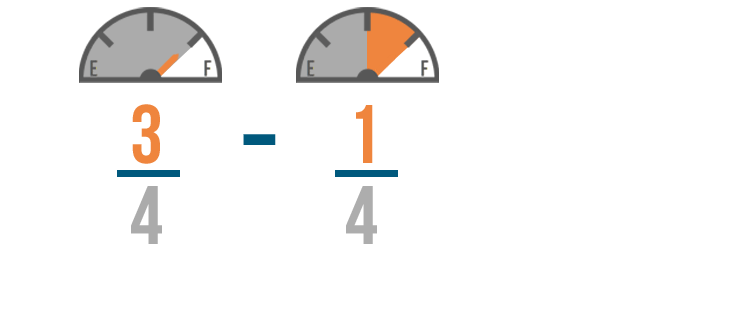

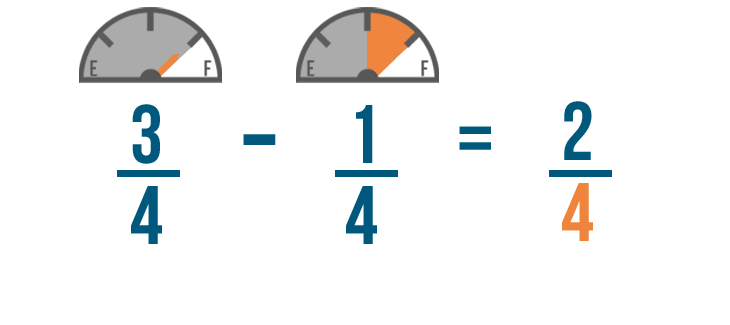
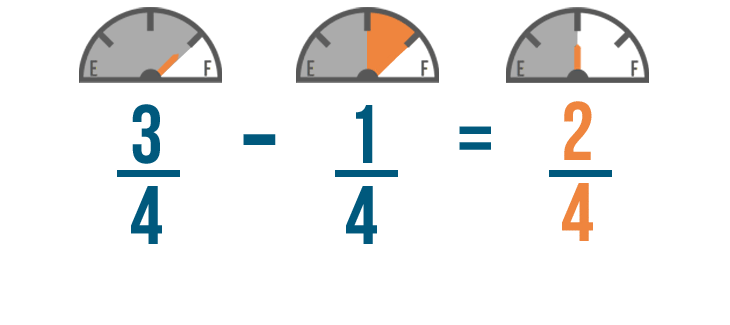
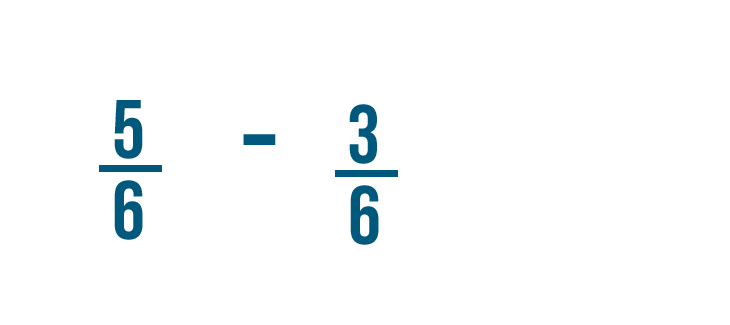
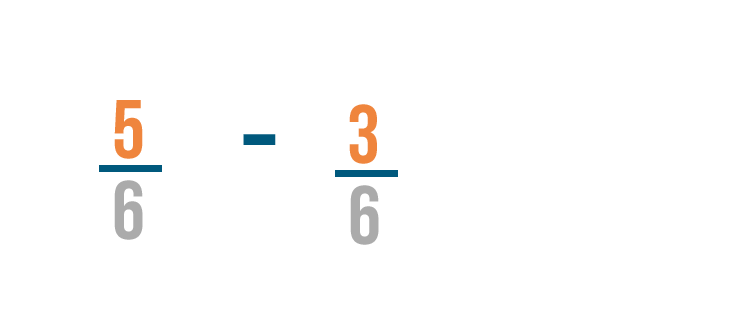
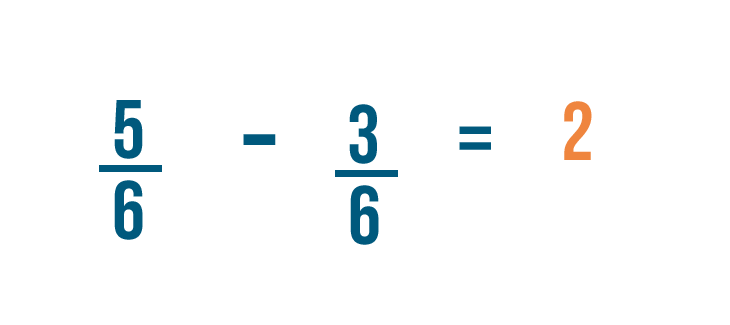

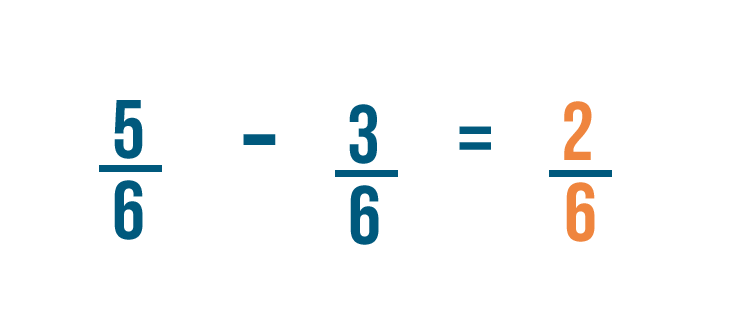

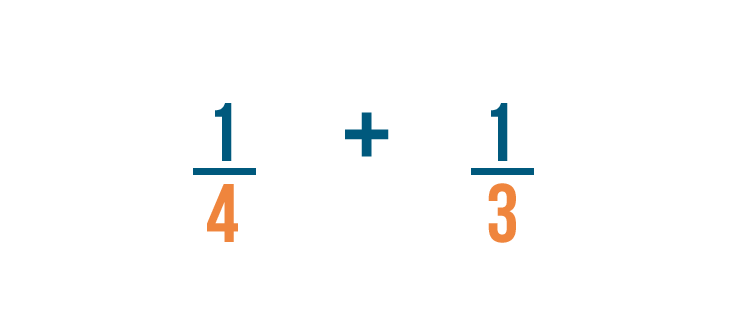

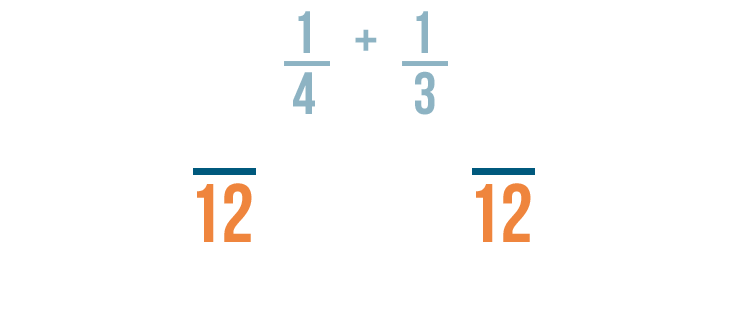
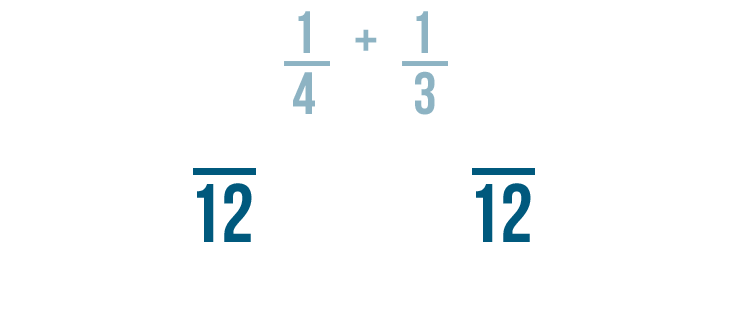
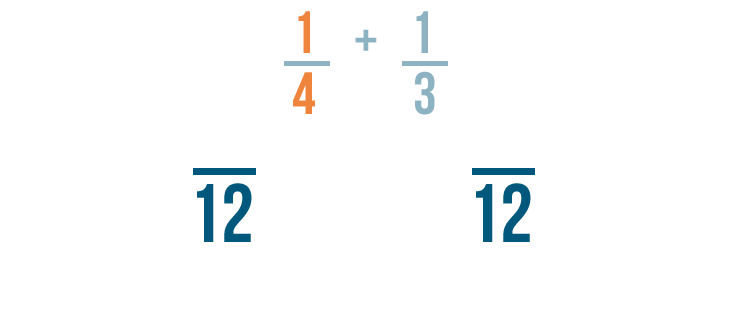
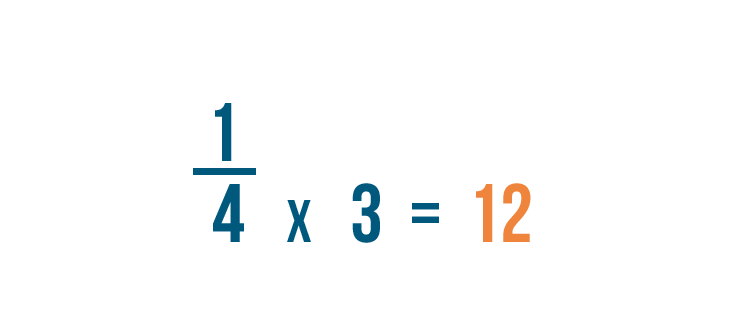
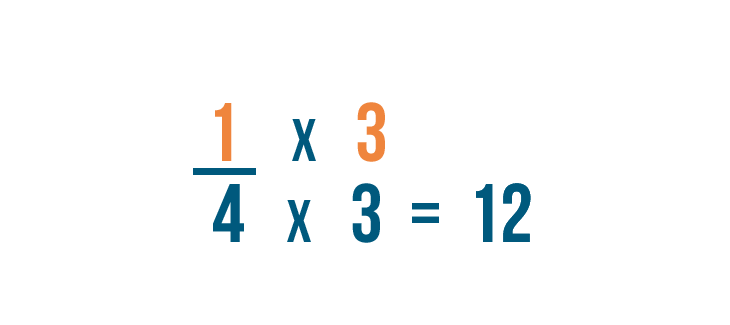
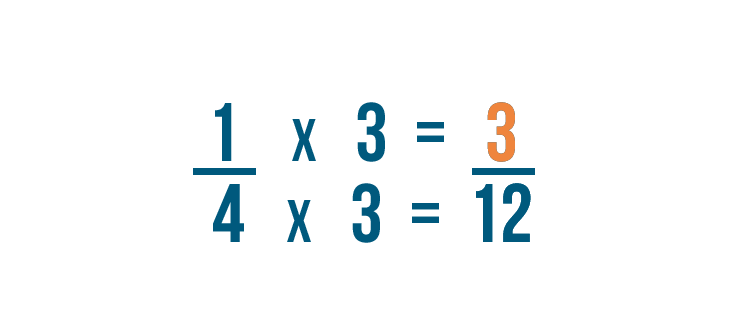
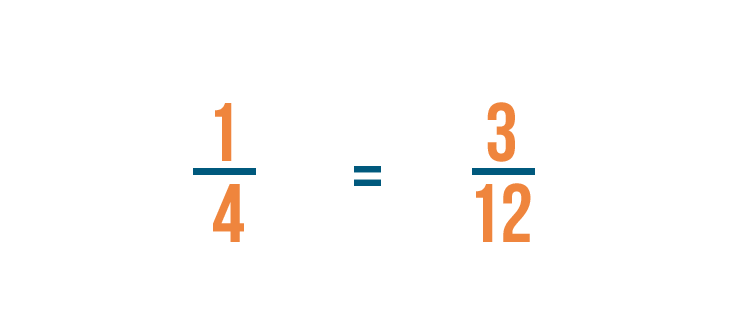
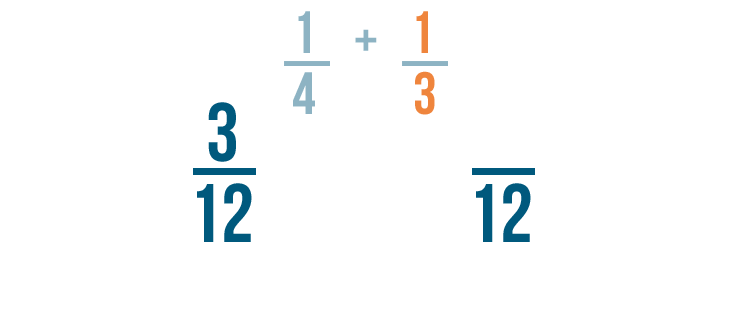
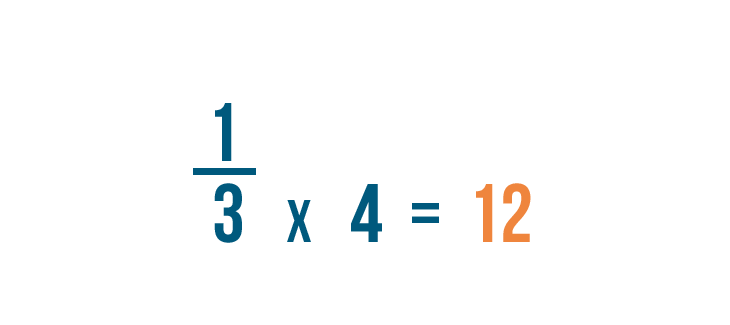

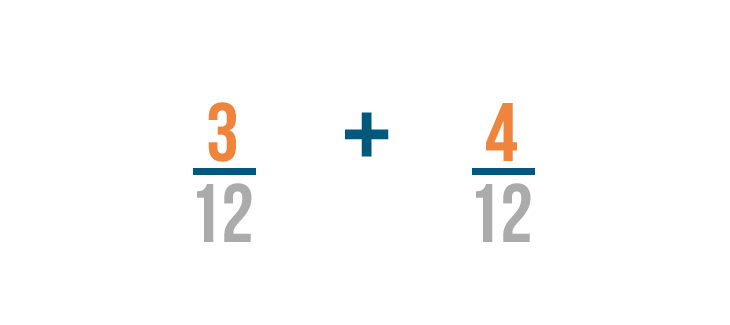
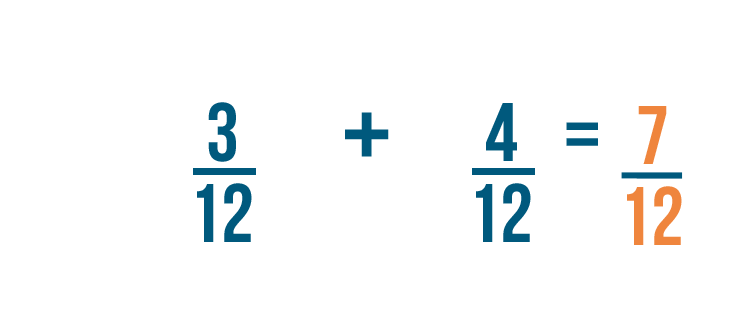
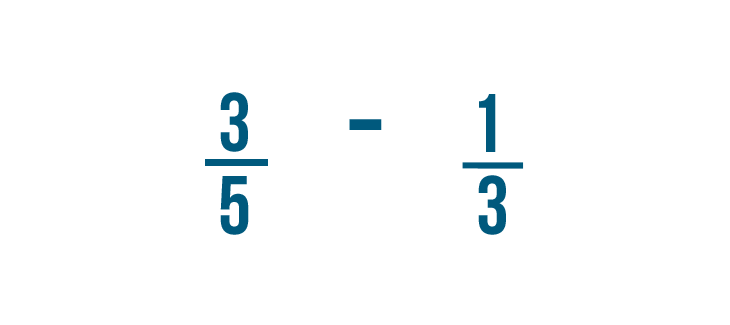
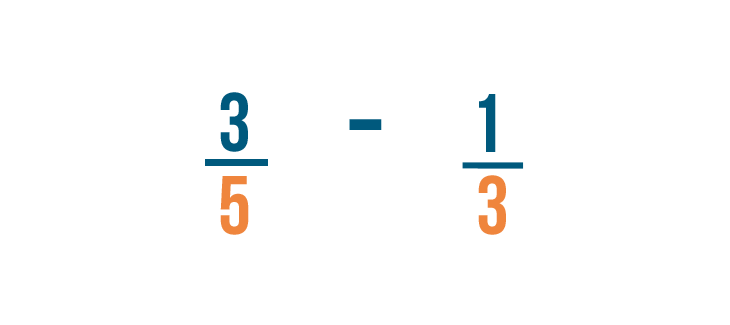


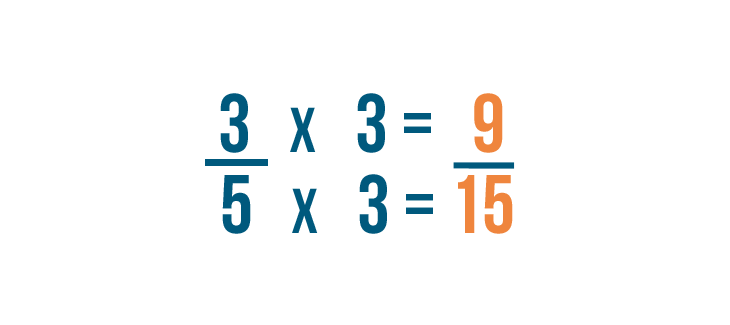

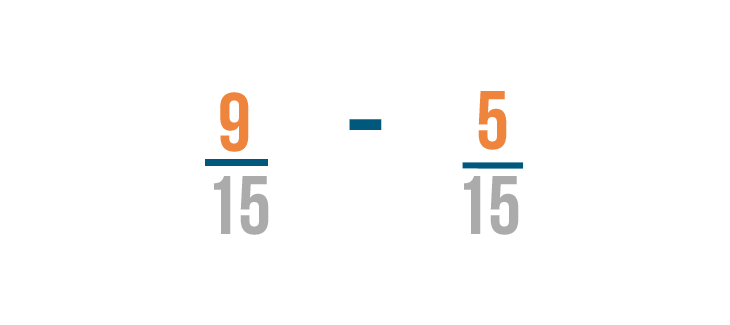


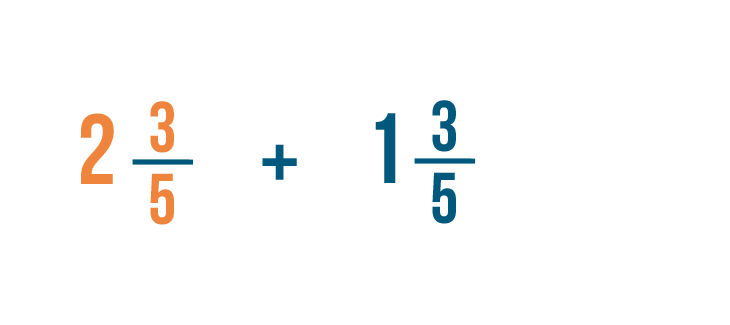
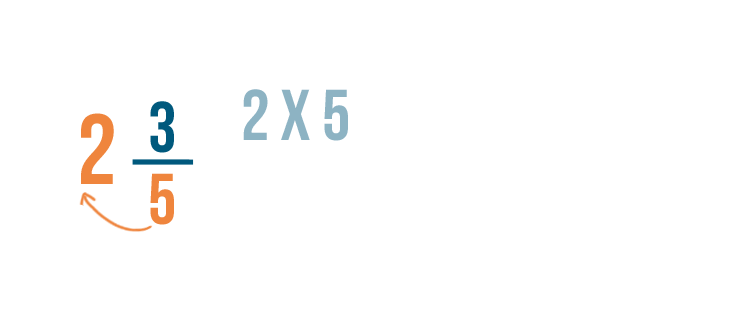

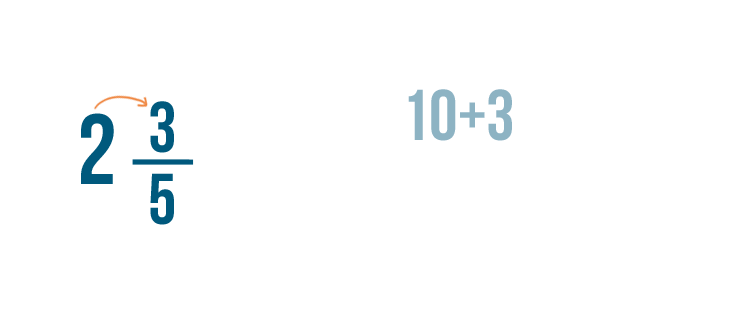
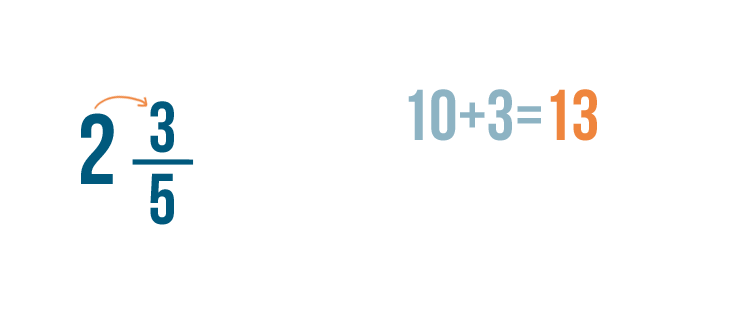

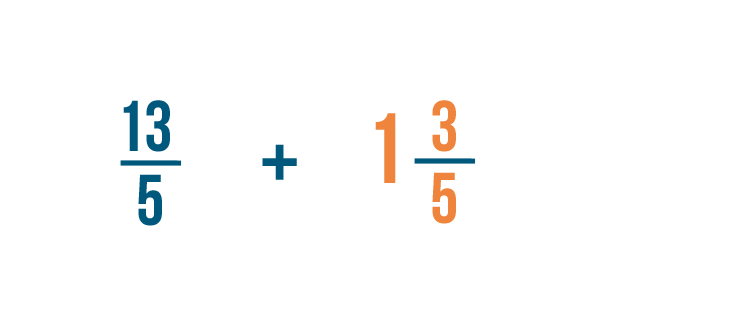

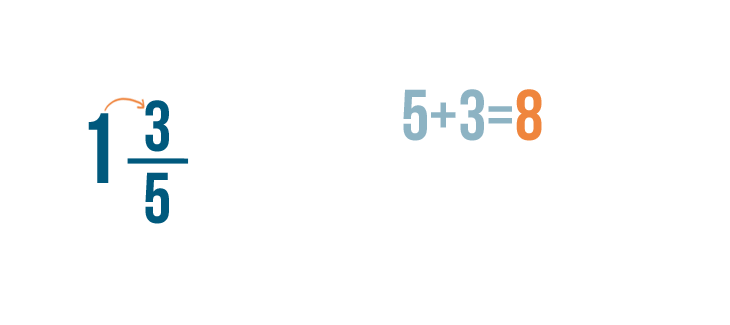

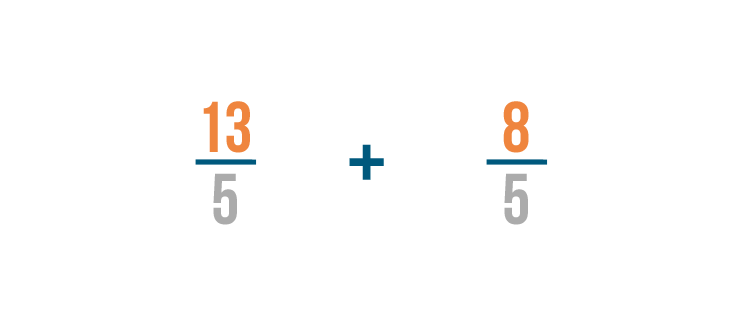
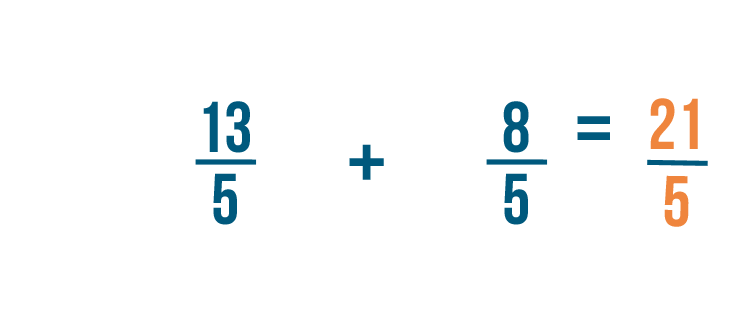
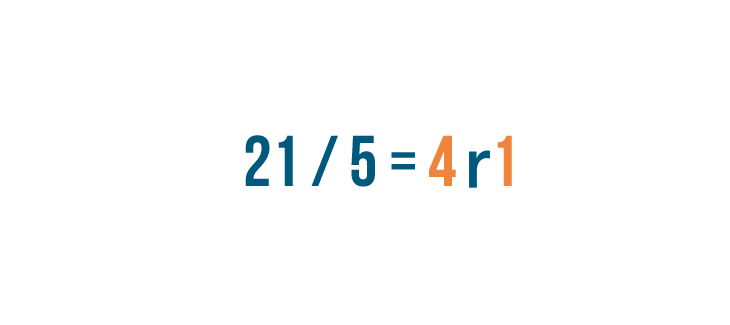
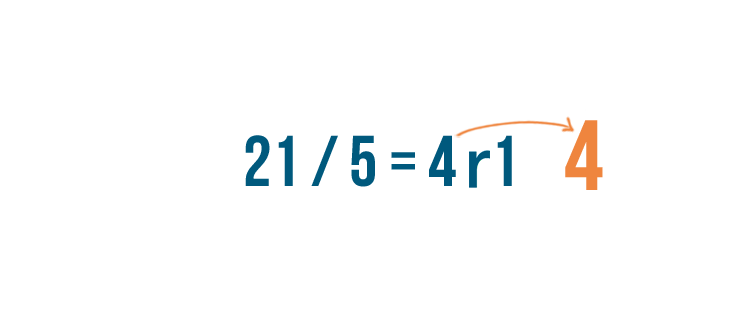
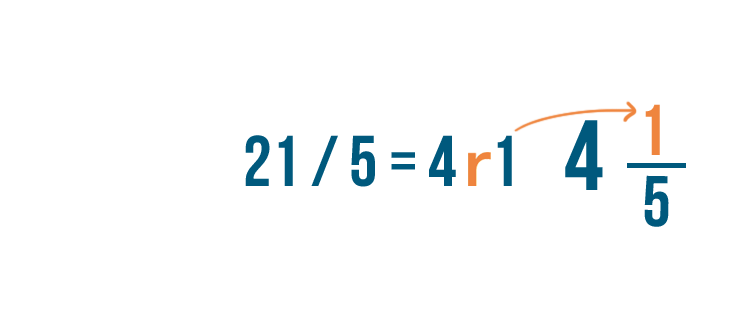
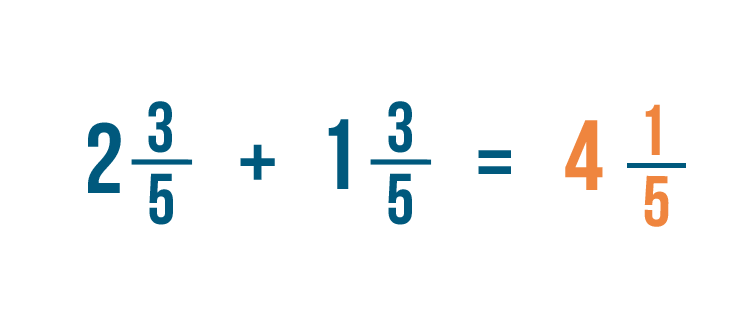
Post a Comment for "Lesson 7 Midunit Review Essential Math 7a Unit 3 Exponents Factors and Fractions Jiskha"